"when George drew out a tin of pineapple from the bottom of the hamper ... we felt that life was worth living after all ... there was no tin-opener to be found ...
I took the tin off myself and hammered at it till I was worn out and sick at heart, whereupon Harris took it in hand.
We beat it out flat; we beat it back square; we battered it into every form known to geometry - but we could not make a hole in it.
Then George went at it, and knocked it into a shape, so strange, so weird, so unearthly in its wild hideousness, that he got frightened."
Jerome K. Jerome
|
Computational
Topology is an
emerging field on the border of mathematics and computer science,
with an important overlap with Computational
Geometry.
The goal of computational
topology proper to GRTCI is to develop an algorithmic and combinatorial
approach to abstract structures of topology with the aim of applying
them in the science and engineering. The applications central to our
interests are those in computer
vision problems, namely, to analysis and reconstruction of digital
images
coming, for example, from medical imaging, to pattern recognition, and
to topological
analysis of multidimensional data. We should emphasize that
computational topology is also applied to many other domains such as
dynamical systems or material science.
Multiple topological
structures are used: cellular complexes, in
particular, cubical and simplicial, homology and homotopy, discrete
Morse theory
and discrete differential geometry. Our first specific goal is to
enhance
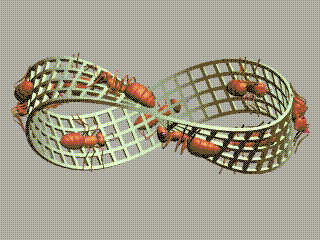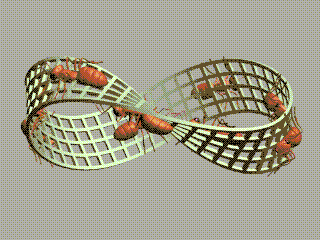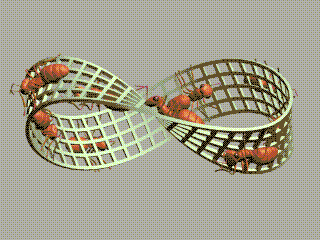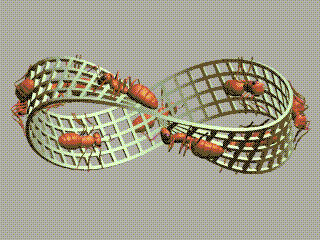
understanding the critical zones in self-intersection of imaged curves
and
surfaces where the mesh generation techniques break due to the
singularity of
the gradient vector field.
Our research project at GRTCI
also aim at interactions between topology and imaging science going the
other way around: computer visualization techniques can improve
understanding topological and geometric structures. Consequently,
they can permit formulating mathematical conjectures to be proved or,
conversely, construct counterexamples to false conjectures.
La Topologie
Computationnelle est un champ d'étude
en émergence situé sur la
frontière des mathématiques et de l'informatique et qui présente un chevauchement important avec la Géométrie
computationnelle.
Un objectif de la topologie computationnelle propre au GRTCI
est de développer une approche algorithmique et combinatoire aux
structures abstraites de la topologie dans le but de les appliquer en
sciences et en génie. Nous nous intéressons surtout aux
applications dans la vision par
ordinateur, notamment : pour l'analyse et la reconstruction des images
numériques provenant, par exemple, de
l’imagerie
médicale; pour la reconnaissance des formes; et pour l'analyse
topologique de
données multidimensionnelles. Il faut noter que la topologie est
appliquée dans plusieurs autres domaines, par
exemple, dans les systèmes dynamiques ou dans la
science des matériaux.
Les structures
topologiques utilisées sont multiples : complexes cellulaires
et, en
particulier, cubiques ou simpliciaux, homologie et homotopie,
théorie de Morse discrète
et géométrie computationnelle discrète. Notre premier but spécifique
est d’améliorer la compréhension des zones critiques dans
l’intersection des
courbes et surfaces, ou des techniques de la génération
de
maillages échouent dû
à la singularité du champ vectoriel gradient.
Les projets de recherche du GRTCI
visent aussi des interactions entre la topologie et l'imagerie
informatique allant dans l'autre sens :
des techniques de
la visualisation par ordinateur peuvent améliorer la
compréhension de structures
topologiques et géométriques, notamment, pour
développer de meilleures
intuitions et lancer des conjectures mathématiques, ou le
contraire, construire des contre-exemples aux conjectures
fausses.
Top page/Haut de page